Quadratic Function and Equation in One Variable

X 3 and is hard to solve so let us graph it instead. Using the below quadratic formula we can find the root of the quadratic equation.

Using The Vertex Formula Quadratic Functions Lesson 2 Graphing Linear Equations Quadratics Vertex

If the quadratic function is set equal to zero then the result is a quadratic equationThe solutions to the univariate equation are called the roots of.

. In addition we introduce piecewise functions in this section. For example a univariate single-variable quadratic function has the form in the single variable xThe graph of a univariate quadratic function is a parabola whose axis of symmetry is parallel to the y-axis as shown at right. The orbit of the critical point.

Y-Intercept Overview Definition. In interval notation we can write. If bb 4ac then roots are complex not real.

The standard form is ax² bx c 0 with a b and c being constants or numerical coefficients and x being an unknown variable. And from the graph we can see the intervals where it is greater than or equal to zero. The nature of roots is determined by the discriminant.

We can define this equation as an equation of second degree or degree of 2. If you have any doubts please comment below. The Standard Form of a Quadratic Equation looks like this.

The general form of the quadratic equation is. The function makes nice curves like this one. Understanding the Discriminant in a Quadratic Formula A quadratic equation in algebra is an equation in which the unknown variables highest power.

We also define the domain and range of a function. What is a Shifting Function Most people have seen some basic graphs before. From 11 to 13 and.

Ax² bx c 0. Here we will learn about the quadratic equation and how to solve quadratic equations using four methods. If given the vertex and one other point on a parabola use the.

Simplification of the above equation gives. It has one finite critical point in the complex plane Dynamical plane consist of maximaly 2 basins. The zero points are approximately.

It is a unicritical polynomial ie. Hope given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 5 Quadratic Equations in One Variable Ex 55 are helpful to complete your math homework. Now figure out which direction the parabola opens by checking.

The standard form of the quadratic equation is ax² bx c 0 where a b and c are real and a 0 x is an unknown variable. A cant be 0. The formula to find the roots of the quadratic equation is known as the.

A quadratic equation is a polynomial equation in a single variable where the highest exponent of the variable is 2. The next example shows how we can use the Vertex Method to find our quadratic function. This means that the highest exponent of the function.

There are three main ways to solve quadratic equations. We also give a working definition of a function to help understand just what a function is. In the equation a b and c are called coefficients.

A quadratic equation is an equation of the second degree meaning it contains at least one term that is squared. For example roots of x2 - 2x 1 are. In this section we will formally define relations and functions.

This is a cubic equation the highest exponent is a cube ie. The name Quadratic comes from quad meaning square because the variable gets squared like x 2. It is also called an Equation of Degree 2 because of the 2 on the x Standard Form.

There are also quadratic equation worksheets based on Edexcel AQA and OCR exam questions along with further guidance on where to go next if youre still. Here x is unknown which you have to find and a b c specifies the numbers such that a is not equal to 0. Hence using the quadratic formula we have x 6 and x -54.

To find the maximum or minimum value of a quadratic function start with the general form of the function and combine any similar terms. Find the inverse function of fleft x right x2 2x ge 0 if it existsState its domain and range. We introduce function notation and work several examples illustrating how it works.

Factorisation using the quadratic equation formula completing the square and using a graph. A quadratic equation is a second-degree equation with one unknown variable. Quadratic equations are the polynomial equations of degree 2 in one variable of type fx ax 2 bx c 0 where a b c R and a 0.

The equation of a parabola is also a quadratic function. Learn Insta try to provide online math tutoring for you. 1 to factor the quadratic equation if you can do so 2 to use the quadratic formula or 3 to complete the square.

For example roots of x2 x 1 roots are -05 i173205 and -05 - i173205 If bb 4ac then roots are real and both roots are same. The vertex form of a quadratic function is eqfx ax-h2 k eq. Quadratic polynomials have the following properties regardless of the form.

X 2 48x -324 0. It is the general form of a quadratic equation where a is called the leading coefficient and c is called the absolute term of f. There is more than one way to find the y-intercept depending on your starting informationBelow are three ways to identify the y-intercept on a graph in a.

In this section first will discuss the quadratic equation after that we will create Java programs to solve the quadratic equation by using different approaches. X is an unknown variable. Quadratics can be defined as a polynomial equation of a second degree which implies that it comprises a minimum of one term that is squared.

A quadratic equation is an equation of the second degree meaning it contains at least one term that is squared. Basin of infinity and basin of finite critical point if finite critical point do not escapes. Speed cant be negative so we have x 6 kmh.

It can be postcritically finite ie. It is also called quadratic equations. One point touching the x.

An example of a Quadratic Equation. What is Quadratic Equation. In the equation ax 2 bxc0 a b and c are unknown values and a cannot be 0.

For example if youre starting with the function fx 3x 2x - x2 3x2 4 you would combine the x2 and x terms to simplify and end up with fx 2x2 5x 4. Keep reading for examples of quadratic equations in standard and non-standard forms as well as a list of. There are following important cases.

If you want to know how to master these three methods. The y-intercept is the point where a graph crosses the y-axisIn other words it is the value of y when x0. What is a quadratic equation.

This same quadratic function as seen in Example 1 has a restriction on its domain which is x ge 0After plotting the function in xy-axis I can see that the graph is a parabola cut in half for all x values equal to or greater than zero. If a 0 then the equation becomes liner not quadratic anymore. Explore the definition and examples of a quadratic function the graph of a quadratic equation when a quadratic.

A b and c are known values. Y-Intercept Sample Questions and FAQs. Lets take an example to solve the quadratic equation 8x 2 16x 8 0.

How to find the y-intercept. Where x is an unknown variable and a b c are numerical coefficients.

Solving Factorable Quadratic Equations A Plus Topper Quadratics Quadratic Equation Equations

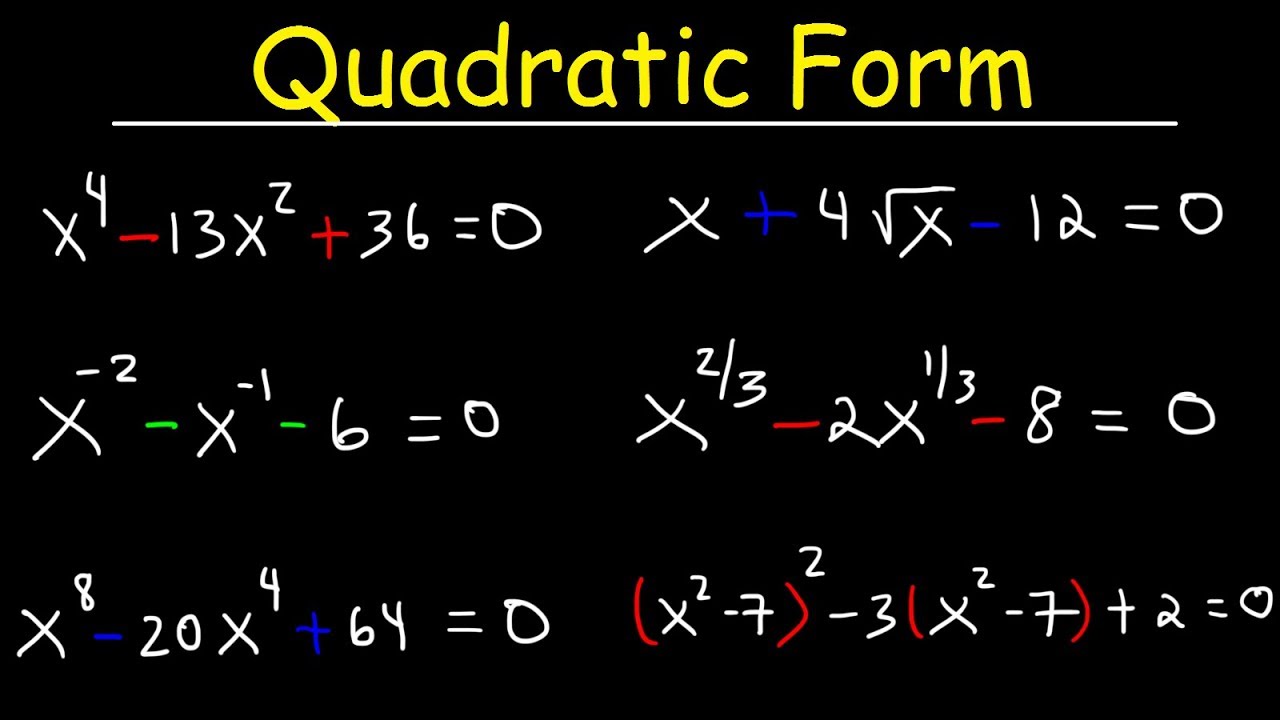
Solving Equations In Quadratic Form Using Substitution Youtube Quadratics Solving Equations Solving Quadratic Equations

How To Solve Quadratic Equations Solving Quadratic Equations Quadratics Quadratic Equation

Quadratic Equation Solving Quadratic Equations Quadratic Equation Quadratics